In any indoor space with boundaries not completely sound absorptive, for example an anechoic chamber used in acoustic laboratories, sound energy will reflect off of the boundaries and back into the space. This is the bases of reverberation: sound reflections continuing to travel after the original sound has stopped. A room mode is a property of those sound reflections. Some sound reflections will reflect off of a boundary and back onto exactly the same path it traveled to the boundary, adding two identical waves will add to each peak and each valley, constructively interfering with itself. This phenomenon increases the amplitude of that wave. When using a space for critical listening it is important that no frequencies are louder than others, and that the reverberation time is as linear as possible.
Sound moves in waves, each different frequency has a different wavelength. Humans can hear frequencies from 20 Hz to 20k Hz. The wavelength of 20 Hz is 56.5 feet long; the length of 20k Hz is 0.678 inches. The wavelength of each hearable frequency is somewhere between those two lengths. To find the wavelength of any way, the following formula is used:
V = velocity
ƒ = frequency
? = wavelength
Velocity depends on the medium in which the wave is traveling. For acoustics the medium is generally air. Although many experiments are carried out underwater, and many vibration isolation projects measure waves traveling through concrete.
The speed of sound traveling through air is 344 m/s or 1128 ft/s at 21 degrees Celsius. The temperature will affect the speed of sound.
For every space, the exact distance between reflective boundaries is the exact size of some wavelength. For example, the wavelength of 1 kHz is 0.344 meters as calculated with the above equation. If the distance between two walls is exactly 0.344 meters, than a single 1 kHz wavelength will fit perfectly between the walls.
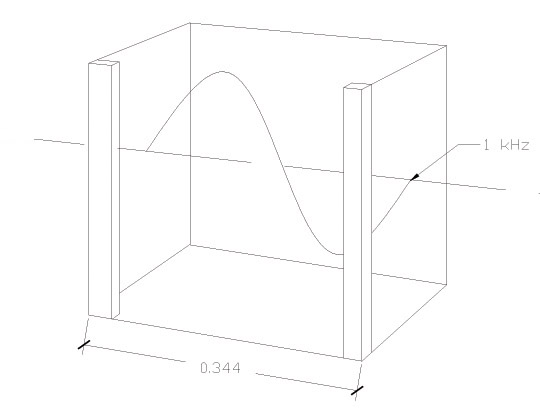
Figure 1 - When the wavelength of a frequency is exactly the distance between boundaries it is called a standing wave.
When the wave fits peak to peak between boundaries, the angle of incidence and the angle of reflection is the same. When this happens the wave bounces back into the room on exactly the same path it took originally. When a wave does this, it interferes constructively with itself, adding to the peaks, and adding to the valleys of the wave.
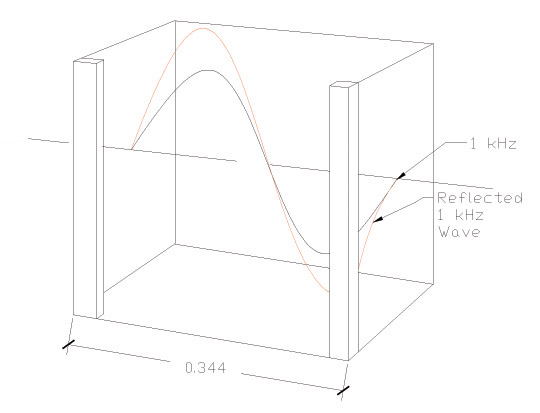
Figure 2 – Due to the same angle of incidence as the angle of reflection, the reflected wave is adding constructively to its peaks and valleys, increasing the amplitude.
When a wave interferes constructively with itself, it gets louder. So if you played each frequency from 500 Hz to 2 kHz all at the exactly same SPL, when playing1 kHz the reflections would make 1 kHz louder than the rest. This happens between all boundaries, between ceiling and floor for example. This is a standing wave, or mode.
In this example, the single mode at 1 kHz is not the only standing wave between these two walls. The wavelength of 2 kHz will be twice that of 1 kHz, therefore two wavelengths will fit perfectly between the two walls. Therefore it will interfere constructively with itself as well as 1 kHz. This will continue at each doubling of frequency.
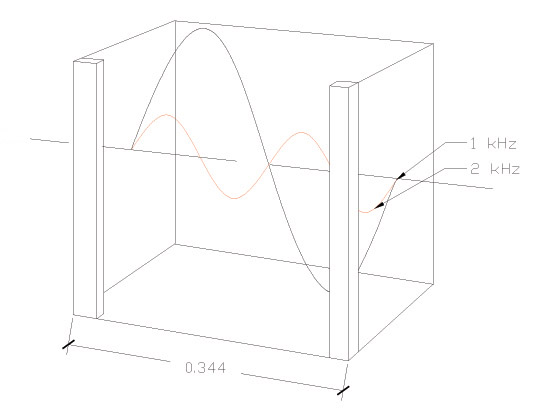
Figure 3 – Any space that perfectly fits a each single 1 kHz wavelength, will perfectly fit two 2 kHz wavelengths, therefore 2 kHz will interfere constructively with itself as well as 1 kHz.
The examples above use 1 kHz only as an example, in real spaces modes higher than 250 Hz do not contribute to a good critical listening environment. Below 250 Hz, the modes color the sound, changing it from the way it’s being played out of the speakers. This is not the only factor creating the way a room ‘sounds’ RT60, speaker placement, background noise, and many other things contribute.
There are three types of room modes: axial, tangential, and oblique. Axial modes occur between two boundaries the example given above is an axial mode, occurring between two walls. Axial modes are the loudest, and the most disruptive to critical listening. Tangential happen between 3 boundaries, between two walls and the floor for example. Tangential modes are potentially disruptive, but most often they do not have enough power to distort the original sound. Oblique modes almost never affect the original playback.
There are going to be room modes in every space, unless a room is an anechoic chamber and absorbs all sound reflections, or unless the space is big enough the distance between all boundaries is larger than a single wavelength of all hearable frequencies. Since room modes are a function of the shape and volume of a space, careful design is needed to control them.
Often used to design critical listening spaces is what is called the “Bonello Criteria”
There is no way to completely get rid of room modes for the reasons described above, therefore the Bonello criterion outlines how room modes should be distributed in a space to make it sound ‘good’. The term ‘good’ for a critical listening application means that we can accurately monitor the playback, that our ears will perceive the sound with no frequencies dominating others.
The Bonello criterion stipulates that in each third octave band, the number of modes should increase smoothly as the frequency is increased. A smooth increase in the number of modes as the frequency increases should give the room a relatively even low-frequency response. The other aspect of the Bonello criteria is that there should be no coincident modes, meaning modes between two different boundaries that occur at the same frequency. There can be coincident modes within a third octave band if there are at least three other non-coincident modes within the same third octave which will help offset the detrimental coincidental modes.
To design a space that will meet the Bonello criteria careful consideration must be used when the room dimensions and surface materials are chosen.